Inter Part II Gujranwala Board 2012
Mathematics Paper: II
Time: 30 Minutes
Marks: 20
OBJECTIVE
Note: You have four choices for each objective type question as A, B, C and D. The choice which you think is correct, fill that circle in front of that question number with marker or pen on the answer book provided. Cutting or filling two or more circles will result in zero mark in that question. Attempt as many questions as given in objective type question paper and leave others blank. Write the letter A, B, C or D in the column (write correct option) against each question. If there is a contradiction in the bubble and hand written answer, bubble option will be considered correct.
(A) y = In x+ c
(B) y = in (cx)
(C) ln y = x+c
(D) xy =c
(A)
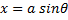
(B)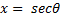
(C)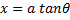
(D)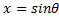
(A)
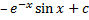
(B)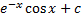
(C)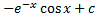
(D)
(A) Maclaurin Series
(B) Taylor series
(C) Binomial Series
(D) Power Series
(A) a > 0
(B) a<0
(C) a<-1
(D) a<-2
(A) 1
(B)-1
(C) 2
(D) 0
(A)

(B)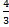
(c)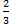
(D)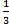
(A) xy1=x1y
(B) xy1=-x1y
(C) xx1+yy1=0
(D) xx1+yy1=a2
(A) n+1
(B) n-1
(C) n
(D) k
(A)
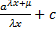
(B)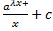
(C)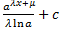
(D)
(A) Zero
(B) I
(C) sec2 x
(D) 2 sec2 x
(A) cos x
(B) sin x
(C)-x
(D)x
(A)
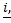
(B)-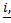
(C) 1
(D)0
(A) (a, 0)
(B) (-a,0)
(C) (0, a)
(D) (0, -a)
(A) Identity function
(B) constant function
(C) linear function
(D) odd function
(A)
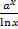
(B)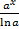
(C)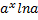
(D)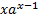
(A)x+y>2
(B)3x+5y> 8
(C)3x+ 7y> 3
(D)3x+5y<8
(A) m=1
(B) m =0
(C) m =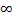
(D) m = -1
(A) — cosh x+c
(B) cosh x+c
(C) ln |cosh x| +c
(D) cosech x + c
Gujranwala Board 2012 Inter part II
Mathematics Paper: II
Time:2.30 Hour Marks:80
SUBJECTIVE
Note: Section I is compulsory. Attempt any three questions from section II (Section-I)
2. Write short answers to any eight questions:3. Write short answers to any eight questions:
4. Write short answers to any nine questions
Section –II
Q.No.5(a) If f(x)={![]()
Find the value of k so that f is continuous at x=2
(b) If x= sin![]() , show that (1-x2)y2-xy1+m2y=0
, show that (1-x2)y2-xy1+m2y=0
Q.No.6 (a) Show that ![]() dx=
dx=![]()
![]() +
+![]()
(b) Find the equation of the perpendicular bisector of the segment joining the points A(3,5) and B (9,8)
Q.No.7 (a) Solve the differential equation y-x![]()
(b) Graph the solution region of the following system of linear inequalities and find the corner points.
2x-3y < 6
2x+3y < 12
x> 0
Q.No.8 (a) Write equation of a circle that passes through the giben points. A(4,5), B (-4,-3), C (8,-3)
(b) The position vectors of points A, B, C and D are 2i-j+k, 3i+j, 2i4j-2k and -i-2j+k respectively.
Show that ![]() is parallel to
is parallel to ![]() .
.
Q.No.9 (a) Prove that the latus rectum of the ellipse:
![]()
(b) Find the area of the triangle with vertices A (1,-1,1), B (2,1,-1) and C (-1,1,2) also find a unit vector perpendicular to the plane ABC
![]()