(12th CLASS - 2014)
MATHEMATICS
CROUP SECOND
OBJECTIVE
TIME: 30 MINUTES,
MARKS: 20
NOTE:- You have four choices for each objective type question as A, B, C and D. The choice which you think is correct, fill that circle m front of that question number. Use mites or pen to fill the circles. Cutting or filling two or more circles will result in zero mark in that question.
Question#1
- Which of the following is an odd function
(A) Coax
(B) Coshx
(C) Sinhx
(D) Sin2
- Lim el/x = ......... , x<0
(A) -1
(B) 0
(C) 1
(D) ∞
- The notion use by Lagrange for derivative is
(A) df/dx
(B) f(x)
(C) f(x)
(D) Df(x)
- If y =
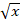 dy/dx
dy/dx
(A) 2,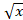
(B) 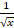
(C) 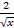
(D) 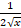
- If f(x) = Sinx , then
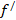 (π) =……
(π) =……
(A)-1
(B) 0
(C) 1
(D) ∞
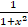 is derivative of
is derivative of
(A) Sin-1x
(B) Sec-1
(C) Tan-1x
(D) Cot-1x
- If y= ex then y3 = ……
(A) ex
(B) e2x
(C) e3x
(D) e-x
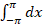
(A) 0
(B) 2
(C) 4
(D) 6
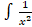 dx =
dx =
(A) Inx +C
(B) Inx2+C
(C) -1/x +C
(D) 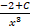
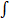 (Cos2 x + Sin2 x) dx =
(Cos2 x + Sin2 x) dx =
(A)Sinx + Cosx+ C
(B) 2Sinx + 2Cosx+ C
(C) -Sinx + Cosx +C
(D) x+C
- The order of differential equation
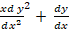 -2=0 is
-2=0 is
(A) 1
(B) 2
(C) 3
(D) 4
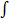 Secx tanx dx =
Secx tanx dx =
(A) sec2 + c
(B) tanx+ c
(C) secx2 + c
(D) None of these
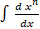 dx =
dx =
(A) 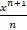 +C
+C
(B) 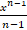 + C
+ C
(C) nx n+1+C
(D) nx + C
- The coordinate axes divide the plane tinto ……………… equal parts
(A) 2
(B) 3
(C) 4
(D) 5
- The centroid of a triangle divides each median in the ratio
(A) 2:1
(B) 1:2
(C) 3:1
(D) 1:3
- A vertical line divides a plane into .. ........... half planes
(A) upper and lower
(B) upper and right
(C) left and right
(D) left end lower
- Asymptotes are very useful in graphing
(A) Circle
(B) parabola
(C) ellipse
(D) hyperbola
- A circle is called a point circle if
(A) r = I
(B) r =0
(C) r = 2
(D) r = 3
- The vector
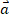 lies in the plane of the vectors
lies in the plane of the vectors 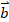 and
and 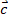 then
then 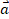 .
. 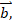 x
x 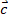 .=………
.=………
(A) 0
(B) 1
(C) – 1
(D) 2
- If a,
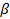 ,
, 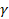 are the direction angles of a vector then Cos2 a + Cos2
are the direction angles of a vector then Cos2 a + Cos2 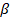 + Cos2
+ Cos2 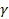 = ........
= ........
(A) 0
(B) 1
(C) 2
(D) 3
(I2th CLASS 1214)
TIME 2.38 HOURS
SUBJECTIVE MARKS: 80
SECTION-I
2. Attempt any eight (8) short questions
- Let f : R→R be the function defined by f(x) = 2x+1 Find f-1 (x)
- Evaluate Lim
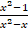
- Find the derivative of x2 w.r.t ‘x' by ab.initio
- Find dy if xy + y2 =2 dx
- Differentiate
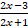 w.r.t ‘x’
w.r.t ‘x’
- Find
 if x = y Sin y dy
if x = y Sin y dy
- If y= in (x2 -9) find y2
- If y = xesinx find dy/ox
- If =
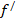 (x) if f(x) =
(x) if f(x) = 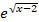
- Find extreme value of f(x) 3x2
- Differentiate Sinx w.r.t Cot x
- Find dy/dx if y = Sinhl (x/2)
3. Attempt any eight (8) short questions . 16
- If xy+x use diffrentials find dy/dx
- Evaluate
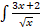 dx
dx
- Evaluate
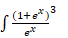 dx
dx
- Evaluate
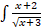 dx
dx
- Evaluate C
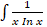 dx
dx
- Evaluate
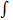 x3 In x dx
x3 In x dx
- Evaluate
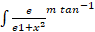 dx
dx
- Evaluate
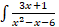 dx
dx
- Evaluate
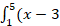 dx
dx
- Solve the differentia equation xdy + y(x-I) dx = 0
- Graph the solution of 2x-3y ≤ 12
- Define the vertex of the solution region
4. Attempt any Nine (9), questions 18
- Find doe coordinates of the point that divides the goal of A (-6.3) and B(5,2) in the I ratio 2:3 internally
- Cheek whether the point (.
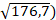 is at a distance of 15 units from origin
is at a distance of 15 units from origin
- Find the slope and indication of the line joining the points (3, - 2) and (2,7)
- Cheek whether the lines, 4y – 2x -1 = 0 and x- 2y -7 = 0 are parallel or perpendicular?
- Find the distance of the point P (6.-1) to the line 6x -4y + 9 = 0
- Find an equation of the circle with none at (
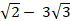 ) and radius 2
) and radius 2
- Cheek the position of the point (5,6) with respect to circle 2x2 + 2y+ 2y2 + 12x -8y + 1=0
- Find IM Focus and vertex of parabola x2 =5y
- Find Foci, eccentricity of ellipse 25x2
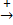 9y2 =225
9y2 =225
- Let A (2,5),B (-1,1), C(2,6) Find
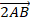 -
- 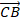
- If u = 2.i + 3j +k , v = 4 I +6 j +2k , w = 06i -9j-3k.. Find | u –v-w|
- Find the projectin of b along a when a = 3i +j –k, b = .2i –j + k
- Find the volume of the parallelepiped determined by u=I +2.j –k,v=i-2j+3k, w=i-7j -4k
SECTION-III
Note: Attempt any three questions from this section
Question#5
(a). Prove that Lim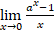 loge a
loge a
(b) Show that y=x has a minimum value at x =1/e
Question#6
(a) Evaluate 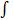 x tan2 dx
x tan2 dx
(b) Find the area Or the triangular n glen whose vertices are A(5.3) ,B(- 2,2) , C(4,2)
Question#7
(a) Solve the differential equation (Siny + y Cosy) dy = [x (2 In,x+1)] dx.
(b) Maximize f (x,y) = 3x+4y≤ 12, 2x +y ≤ 4, 4x -y ≤4x≥ 0 , y ≥0
Question#8
(a) Find equation of circle passing thmugh A(3,- I) , B(0,I) and having centre at 4x - 3y - =0
(b) Prove Cos (a + 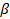 ) = Cos a Can
) = Cos a Can 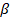 - Sin a Sin
- Sin a Sin 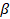 by vectors raethod
by vectors raethod
Question#9
(a) Find the equation of parabola Eying focus at (2,5) and directix y =1
(b) Find the area of thc triangle by -.actor method if 1(0,0,0), Q(2,32) ,12(- l,),41