Inter part Gujranwala Board 2014
Statistics (New Scheme)
Paper I (Objective)
Time: 20 Minutes
Marks: 17
Note: You have four choices for each objective type question as A B, C and D: The choice which you think is correct, fill that circle in front of that question number with marker or pen on the answer book provided. Cutting or filling two or more circles will result in zero mark in that question Attempt as  many questions as give; in objective type question paper leave other blank.

Question#1

• For positively skewed distributions 
  
  (A) Mean < median < mode
  (B) Mean > median > mode
  (C) Mean < mode < median
  (D) Mean = median = mode
  
  
• If A and B are two dependent events then.
  
  (A) P(AUB)= P(A).P(B)
  (B) P(A∩B) = P(A).P(B/A)
  (C) P(A/B =P(A)
  (D) P(A∩B) = P(A).P(B/)
  
  
• The hyper geometric distribution has__________parameters.
  
  (A) 0
  (B) 1
  (C) 2
  (D) 3
  
  
• The variance expresses the variability of data in________ as 'unit of data.
  
  (A) Square of unit
  (B) Square root of unit
  (C) Same unit 
  (D) All of these
  
  
• Statistics is a science of
  
  (A) Decision making
  (B) Averages.
  (C) Collection of data 
  (D) estimates
  
  
• Variance of binomial distribution is
  
  (A) N
  (B) Np
  (C) Npq
  (D) Obj
  
  
• In a discrete probability distribution, the sum of all the probabilities is always
  
  (A) 0
  (B) 1
  (C) -1
  (D) ∞
  
  
• If. A and B are two mutually exclusive events then
  
  (A) P(AUB)= P(A).P(B)
  (B) P(AUB) = P(A).P(B)
  (C) P(A∩B =P(A).P(B)
  (D) P(A∩B) = P(A).P(B)
  
  
• Var (bx)= _________
  
  (A) b var (x)
  (B) b2 var (x)
  (C) var (x)
  (D) var( x)Obj 
  
  
• Geometric mean for x1 and x2 is
  
  (A)     +
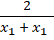
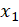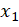
  (B)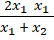 
  (C)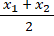   
  (D)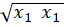      
  
                   
  
• In a frequency distribution, the total of class frequencies is
  
  (A) 1
  (B) 100
  (C) ∑f 
  (D) ∑x
  
  
• The reciprocal of arithmetic mean of the reciprocals of observations is called
  
  
  (A) Mean
  (B) Median
  (C) Mode
  (D) H.M
  
  
• Binomial distribution is negatively skewed if
  
  (A) p <
  (B). p >q
  (C) p=q
  (D) np = npq
  
  
• Consumer price index is obtained by
  
  (A) Paache's formula               
  (B) Fisher's ideal formula
  (C) Marshall Edgeworth formula
  (D) Family budget method formula      
  
  
• An expected value of a random variable is equal to its
  
  (A) Variance
  (B) S.D.
  (C) Mean
  (D) Covariance
  
  
• If all the value of are equal importance, the index number is called 
  
  (A) Weighted 
  (B) Unweighted
  (C) Composite
  (D) Value index
  
  
• The sum a devotions is always equal to zero, when deviations are taken from
  
  (A) Mean
  (B) Median
  (C) Mode
  (D) G.M
  

Inter (Part-I)
Gujranwala Board 2014
Statistics (New Scheme)
Paper: I(Subjective)
Time: 3:10 hours
Marks: 83
(Section I)
Note: (Section I) is compulsory. Attempt any three (3) question from Section II and any three parts from Section III.

2. Write short answers to any EIGHT questions: (2x8-16)

• Define inferential statistics.
  
• What is primary data?
  
• If Mode 50„ Median = 12, find Mean.
  
• Find A. M. if U = x-57/5 , ∑U = 23, n = 20
  
• Define weighted mean.
  
• If ∑(x- 15) = 5, ∑(x - 18) =0. ∑(x - 21)= -21,-what is the value of mean and why?
  
• What are the merits and demerits of median (any two)?
  
• What is importance of C.P.I ?
  
• Define Laspeyre's index number.
  
• Define weighted index numbers.
  
• What is current period?
  
• What are the limitations of index numbers?

3. Write short answers to any EIGHT questions:(2*8=-16)

• Define frequency distribution.
  
• Define simple bar diagram.
  
• Define dispersion,
  
• Define co-efficient of variation.
  
• Find the co-efficient of mean deviation, given ∑│X-X.│= 20.404, n = 5 and ∑X 457.08
  
• Define skewness.
  
• Given mean =100, mode = 95 -and S.D. = 10, find co-efficient of skewness.
  
• If Var(X) = 5, find Var(2X+ 10)
  
• Define random experiment.
  
• Define mutually exclusive events,
  
• If P(A)- 0.5 and P  = 0.3 find P(A ∩ B)
  
• A fair die is rolled. Find the probability that an odd number appears.

 4. Write short answers to any SIX questions: (2*6 = 12)

• Define random variable.
  
• What is mathematical expectation of a random variable?
  
• What is random experiment?
  
• Write down any two properties of expected values.
  
• How is random number table generated?
  
• What are the parameters of binomial distribution?
  
• Define binomial experiment.
  
• Give two properties of hypergeometric experiment which are different from binomial experiment.
  
• Find the mean of hypergeometric distribution when N = 8,n = 2 and K = 8,

(SECTION-II)

Question#5
(a) A variable is obtained from a variable x by the equation y =10 - 2x. Determine the y values when x values are: x= -3, -2, -1, O. I, 2, 3, 4, 5 and show that  y 10 - 2 x
(b) Find the geometric mean from the following frequency distribution:     

Question#6
(a) Calculate mean deviation from mean and its co-efficient for the following data: 4,   10, 7, 9, 15, 14, 8, 10, 7, 11
(b) For the following data find combined standard deviation and combined co-efficient of variation:
n₁ = 50, x₁ = 63,S₁ = 9
n₂= 40, x₂ = 54,S₂= 6

Question#7
(a) Compute price relatives for the following data taking 2001 as base year:

(b) In a poker hand consisting of 5 cards. What is the probability of getting,
(i) an ace 
(ii) 5 spades

Question#8
(a) A continuous random variable x having value only between 0 and 4 has density function given by f(x) = ½ - ax where a is constant.Find
(i) a 
(ii) P(1 < x < 2)
(b) From the following probability distribution.

Verify that E(2X +3) = 2E(X) + 3

Question#9
(a) A fair coin is tossed 4 times. Find the probability
(i) 4 heads
(ii) One tail and 3 heads
(iii) At least 2 heads
(iv) At the most 2 heads
(b) A committee of size 3 is selected from 4 men and 2 women, Find the probability distribution by hypergeometric experiment for the number of men on the committee.

(SECTION-III)
(Practical)

Question#10
(A) Find median from the 

 (B) Find M.D. from the following:

(C) Find Index Number by Chain Base Method:

(D) Find Mean, Variance and E(3x - 2) from the following Probability distribution.

(E) An event has the probability P= 2/3. Find the complete binomial distribution for n = 5. Find mean and variance of probability distribution.