Inter (Part-II) Gujrawala Board 2015
Mathematics
Part I (Objective Type)
Time Allowed: 30 Minutes
Max. Marks: 20
Note: You have four choices for each objective type question as A, B, C and D. The choice which you think is correct; fill that circle in front of that question number. Use marker or pen to fill the circles. Cutting or filling two or more circles will result in zero mark in that question.
Question #1
Circle the correct option i.e. A/B/C/D. Each part carries one mark.
(a)

(b)
(c)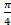
(d) π
(a) ex cosx
(b) ex sinx
(c)-ex sinx
(d)-ex cosx
(a)cosec2x
(b)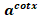
(c)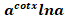
(d)
(a) lnsin2x
(b)(lnsinx)2
(c)(lnsinx)2
(d) none
(a) -cosec2x
(b) –cotx cosecx
(c)ln cos x
(d) ln sin x
(a) f" (c)=0
(b) f" (c) < 0
(c) f" (c)0
(d) f" (c) > 0
(a) cos x
(b) sin x
(c)n(l+x)
(d)
(a)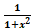
(b)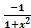
(c)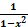
(d)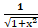
(a) cot x
(b) tan x
(c) -cot θ
(d) -tan θ
(a) -sin 2 π
(b)-2 sin 2 π
(c) 0
(d) 1
(a) 1
(b) 0
(c)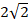
(d)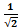
(a) R
(b) (-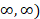
(c) (0,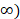
(d) [-1, 1]
(a)

(b)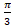
(c)
(d)
(a)
.
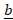
(b).
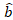
(c).
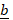
(d).
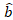
(a)x=2
(b)x = -4
(c)y=2
(d) y = -2
(a) (± 4, 0)
(b) (0, ± 4)
(c) (0, ± 2)
(d)(± 2, 0)
(a) (1, 1)
(b) (1, 3)
(c) (1, 4)
(d) (1, 5)
(a)π
(b)
(c)
(d)
(a) c = 0
(b) a = 0
(c) a=b
(d) b =0
(a) 4
(b) 3
(c) 2
(d) 1
Inter (Part-II) Gujrawala Board 2015
Mathematics
Part I (Subjective)
Time Allowed: 2.30 Hours
Max. Marks: 80
Section I
Q2.write short answers to any eight questions: (2*7=16)
Q3. Write short answers to any EIGHT questions: (2x8=16)
Q4. Write short answers to any NINE questions: (2x9=18)
(Section-II)
Question #5
(a) Evaluate the limit ![]()
(b) If y = x4+ 2x2+ 2, prove that ![]()
Question #6
(a) Evaluate ![]()
(b)Find the equation of line through the intersection of two lines x+ 2y + 3 = 0 and 3x+4y+ 7 = 0 and making equal intercept on the axes.
Question #7
(a) Evaluate ![]() tdt
tdt
(b) Maximize z = x + 3y Subject to 2 x + 5y ![]() 30
30
5x + 4y ![]() 20
20
x ![]() 0, y
0, y![]() 0
0
Question #8
(a) Find the equations of tangents to the circle x2 + y2 = 2 parallel to the line x - 2y + 1 = 0
(b) Prove that the line segment joining the mid - points of two sides of a triangle is parallel to third side and half as long.
Question #9
(a) Find focus, vertex and directrix of parabola x2- 4x - 8y + 4= 0.
(b) Use vector method to prove sin (![]()