RAWALPINDI BOARD 2015
PAPER STATISTICS PART-I
Time: 20 Minutes
(Objective Part) 
Marks:17
Note: Four Answers are given against each column A,B,C&D, Select the write answer and only separate answer sheet, fill the circle A,B,C or D with pen or marker in front of that question number.

• The price relatives computed by chain base method are called:
  
  (a) Price relatives
  (b) Chain indices
  (c) Link relatives
  (d) None of them
  
  
• Index number for base period always taken as:
  
  (a) 1000
  (b) 100
  (c) 200
  (d) Zero
  
  
• The probability of getting 7 in throwing a dice is:
  
  (a) 117
  (b) 1/6
  (c) 0
  (d) 1/3
  
• If P(B/A)41.5 and P(4 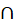 B) = 0.4 then P(A) will be equal to:
  
  (a) 0.40
  (b) 0.5
  (c) 0.80
  (d) 1
  
  
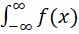•  is always equal to
  
  ((a) Zero
  (b) One
  (c) E(x)
  (d) t(x)+1
  
  
• The probability function of a random variable is defined as:
  

  X	-1	-2	0	1	2
  f(X)	K	2K	3K	4	5K

  Then K is equal to:
  
  (a) Zero
  (b) One
  (c) 1/15
  (d) 1/5
  
  
• In binomial experiment, the trails are:
  
  (a) Constant
  (b) Independent
  (c) Dependent
  (d) None of these
  
  
• If n =10 and P = 0.5 then mean of binomial distribution,
  
  (a) 10
  (b) 5  
  (c) 0.5
  (d) 0.25
  
  
• Hypergeometric distribution become binomial distribution when:
  
  (a) N=n 
  (b)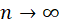
  (c)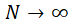
  (d) N < n
  
  
• The life time of TN tube is a:
  
  (a) Discrete variable
  (b) Continuous v able
  (c) Constant
  (d) Qualitative variable
  
  
• The group data are called
  
  (a) Primary data
  (b) Secondary data
  (c) Discrete frequency distribution
  (d) Difficult to tell
  
  
• If the number of workers in a factory is 256 then the number of classes will be:
  
  (a) 8
  (b) 9
  (c) 10
  (d) 12
  
  
• If 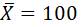and Y = 2x - 200 then  will be:
  
  (a) 2
  (b) 0
  (c) 100
  (d) 200
  
  
• If 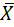 = 25 which of the following will he least?
  
  (a)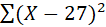  
  (b)
  (c)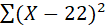  
  (d)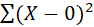
  
  
• The 1st moment about X = 0 of a distribution is 12.08 then mean is:
  
  (a) 10.80
  (b) 10.08
  (c) 12.08
  (d) 12.88
  
  
• The variance of -5, -5, -5, -5 is:
  
  (a) 5
  (b) 0
  (c) -5
  (d) 4
  
  
• The mean deviation of series 2,4,6, is:
  
  (a) 0
  (b) 1.33
  (c) 4
  (d) 12
  

Time: 2:40 Hours
Subjective Part
Marks:68
SECTION-I

2. Write short answers of any Eight Parts. 16

• What is statistics?
• Define secondary data.
• What is quartile?
• Define mode.
• Define arithmetic mean.
• What is the sum of deviations from mean?
• Write any two merits of geometric mean.
• Define composite index number.
• What is link relative?
• Write down the formula for household budget method.
• What is consumer price index number?
• What is the name of Paasche's index number?

3. Write short answers of any Eight Parts. 16

• What is histogram?
• Name any four parts of a statistical table.
• What is relative dispersion?
• What are the names of measures of absolute dispersion?
• Find the range of -1, -3, 0, 2, 3
• What is mean deviation?
• Differentiate between inter quartile range and quartile deviation.
• What are values of 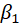 and 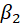 in a symmetrical distribution?
  
• What are combinations?
  
• What is a sample space?
  
• Give the addition law for two mutually exclusive events.
  
• What are exhaustive events?

4. Write short answers of any Six Parts

• Define in,hematical expectation.
• If E(X) =2.5 and E(x²)=9.25 find var(x)
  
• How random numbers are generated?
  
• Given E(X) = 204 and C.V_(x) =6 find S.D_(x)
  
• Define distribution function.
  
• What is binomial distribution?
  
• Define hyper geometric experiment.
  
• In binomial distribution if n = 10 and p=¼ then find variance of binomial distribution.
  
• Write down any two properties of hyper geometric distribution.

SECTION-II:
Attempt any Three questions. Each questions carries 8 marks.

Question#5
(a) Following data have been obtained from a frequency distribution of a continuous variable 'x' after making the substitution
U= 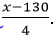. Find G.M. of the variable `x'.

(b) Find mode:

Question#5
(a) Calculate the mean deviation for the data given below:

(b) Th four moments about x=62 are 1,4,10 and 16. Find first four moments about mean.
Question#5
(a) Given the prices of rice in Sargodha in rupees per quintal from 1987 to 1992. Calculate the simple index numbers with 1987 as base year.

(b) A digit i selected at random from the first 10 natural numbers. Find the probability that the selected digit is:
(i) Great that 6
(ii) A complete square
(iii) Multiple of 3
Question#5
(a) Find the coefficient of variation from the following probability distribution:

(b) A continuous random variable x has a density function
f(x) = 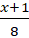 for x =2 to x = 4, Find:
(i) P ( x < 3.5)
(ii) P(2.4 £ x £ 3.5)
Question#5
(a) If 13 cards are chosen at random (without replacement) from an ordinary deck of 52 cards find the probability that 6 are picture cards.
(b) In a binomial distribution, the mean and the standard deviation were found to be 4.5 and 1.5 respectively. Find n and p.

SECTION-III
NOTE: Attempt any Three questions.

Question#10
(A) A variable 'Y is determined from a variable 'X' by the equal Y = I0-4X.
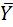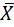Find 'Y' when X = -3, -2, -1, 0, 1, 2, 3, 4, 5 and show that =10-4.5
(B) By using provisional mean, calculate variance for the values 14, 16, 23, 25, 29, 30, 35, 40.
(C) Given the following information:
∑p₀q₀=3600,∑p₁q₀, = 4300 ,∑p₀q₁ =4100, ∑p₁q₁ = 4890.  Show that Fisher's Ideal Price index number is the G.M of Laspeyre's and Paasche's index number.
(D) Let 'X' has the following probability distribution:

Find E(X) and E (X²)
(E) Four dice are tossed 96-times. Find the expected frequencies throwing of 4, 5, or 6 is regarded as a success.