Bahawalpur Board 2017
INTERMEDIATE PART-II (12th CLASS)
Mathematics (NEW SCHEME)
TIME ALLOWED: 20 Minutes
MAXIMUM MARKS: 15
OBJECTIVE
Note: You have four chokes for each objective type question as A, B, C and D. The choice which you think is correct; fill that circle in front of that question number. Use marker or pen to fill the circles. Cutting or filling two or more circles will result in zero mark in that question. Attempt as many questions as given in objective type question paper and leave others blank. No credit will be awarded in case BUBBLES are not filled. Do not salve question on this sheet of OBJECTIVE PAPER.
Q.No.1
(A) Domain
(B) Range
(C) Function
(D) Dependent Variable
(A) e-1
(B) e
(C) e2
(D) e3
(A) n (axn-1+ b)
(B) n (ax+ b)n-1
(C) naxn-1
(D) na (ax + b)n-1
(A)
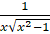
(B)
(C)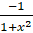
(D)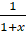
(A) e2x
(B) 4e2x
(C) 2e2x
(D) 8e2x
(A) Sin h x
(B) -Sin h x
(C) Cosec h x
(D) Cot h x
(A)

(B)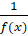
(C)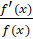
(D) f (x) . f’ (x)
(A) -2Sin2x + C
(B) 2Sin2x
(C) -

(D)
+ C
(A) ex-Cos x
(B) ex + Sin x + C
(C) ex + Cos x + C
(D) Cos x + C
(A)
+ C
(B)+ C
(C)
(D) ax, Ina + C
(A) In (ex+2) +C
(B) In (ex + 3)
(C) In (ex - 2) + C
(D) In (ex- 3) + C
(A) (x2- x1)²+ (y2 – y1)²
(B)
(C)
(D)
(A)
(B)
(C)
(D)
(A) y = mx + c
(B) y + y1 = m(x + x)
(C) y + y1 = m (x + x1)
(D) y –y1 = m (x – x1)
(A) ax - by + c = 0
(B) ax + by - c = 0
(C) ax + by + c = 0
(D) ax - by- c = 0
(A) Four Variable
(B) Three Variable
(C) One Variable
(D) Two Variable
(A) (1, 1)
(B) (0, 0)
(C) (0,1)
(D) (1, 0)
(A)
(B)
(C)
(D) 2ab
(A)
+2
+ 3
(B)+2
+ 3
(C)-2
-3
(D)-2
-3
(A) -1
(B) 0
(C) 1
(D) -2
Bahawalpur Board 2017
INTERMEDIATE
PART-II (12th CLASS)
Mathematics (NEW SCHEME)
TIME ALLOWED: 2.10 Hours
MAXIMUM MARKS: 60
SUBJECTIVE
SECTION-I
1. Attempt any Eight of the following. All carry equal marks.
3. Write short answers to any Eight questions.
4. Write short answers to any Nine questions.
(SECTION II)
5. (a). Prove that ![]() =
=![]()
(b). If x=Sin, y=Sin m![]()
6. (a). Evaluate ![]()
(b). Find a joint equation of the Straight Lines through the origin perpendicular to the lines represented by x²+ xy - 6y²= 0
7. (a) Evaluate ![]()
(b) Minimize f(x , y) = 2x +y Subject to the Constraints x +y ![]() 3 7x+5y
3 7x+5y ![]() 35; x
35; x![]() 0
0
8. (a) Write an equation of the Parabola with given elements Focus (-3 , 1) and Directrix x = 3
(b) Show that Mid-Point of Hypotenuse a right triangle is equidistant from its vertices.
9. (a). Write Equation of the Tangent to the give conic at the indicated point. 3x² = -16y at the points whose ordinate is -3.
(b). Find Volume of the Tetrahedron whose vertices are A(2 , 1 , 8) , B(3 , 2 , 9) , C(2, 1, 4) and D(3 , 3, 10)